The aritmetic sequence has the characteristic that each term is the term before plus a constant number. Then we can create the following system of equations:
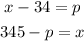
where p is the constant value which is added in each term, and x the number between 34 and 345. If we replace the value of "x" from the second equation into the first one:
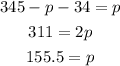
Finally, the term betwen 34 and 345 is 34+p, 189.5
Hence the answer is 189.5