
1) The best way to tackle questions like these is to sketch out:
2) We were told that this is an isosceles triangle therefore at least 2 of their angles are congruent to themselves. Therefore we can write down the following equation also considering the Triangle Sum Theorem:
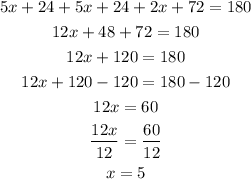
Note that now, we can find the measure of each angle by plugging x=5:
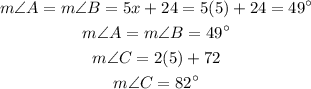
3) Thus the answer is:
