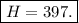Answer:
397 meters
Step-by-step explanation:
Let us draw a diagram to understand the situation better.
Where H is the height of the plane.
Now the tan ratio for 5° gives

Since opposite = H and adjacent = 4535 meters, the above gives
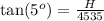
Multiplying both sides by 4535 gives
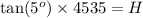
Since tan(5°) =0.0875.., ( just used a calculator to find out) the above becomes
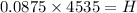
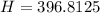
rounding to the nearest meter gives