Question

A vector has a magnitude of 50 and a direction of 30°. Another vector has a magnitude of 60 and a direction of 150°. What are the magnitude and direction of the resultant vector? Round the magnitude to the thousandths place and the direction to the nearest degree.

Answer 1

Step 1:

Draw the vector diagram

Step 2:

Write the angles to the horizontal axis.

30 degrees to the horizontal axis = 30

150 degrees to the horizontal axis = 180 - 150 = 30

Step 3:

Find the vertical component and the horizontal component of the magnitude.

`\begin{gathered} \text{Horizontal component = Fcos}\theta \\ \text{Vertical component = Fsin}\theta \end{gathered}`

Step 3:

`\begin{gathered} \text{Sum of the vertical component V = 25 + 30 = 55} \\ \text{Sum of the horizontal component H = 43.3 - 51.96 = -8.66} \end{gathered}`

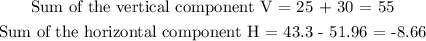
Step 4:

Find the magnitude

`\begin{gathered} \text{Magnitude = }\sqrt[]{V^2+H^2} \\ =\text{ }\sqrt[]{55^2+(-8.66)^2} \\ =\text{ }\sqrt[]{3025+74.9956} \\ =\text{ 56.678} \end{gathered}`

Magnitude = 56.678

Step 5:

Find the direction

`\begin{gathered} \text{Tan}\theta=\text{ }(V)/(H) \\ \theta=tan^(-1)((55)/(8.66)) \\ \theta\text{ = 81} \end{gathered}`

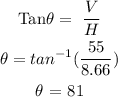
Direction = 81