Question

Graph for 6x - 2y > -11

Answer 1

To graph the inequality:

`6x-2y>-11`

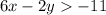
We use the x and y-intercepts.

When x=0

`\begin{gathered} 6x-2y>-11 \\ 6(0)-2y>-11 \\ -2y>-11 \\ (-2y)/(-2)<(-11)/(-2) \\ y<5.5 \end{gathered}`

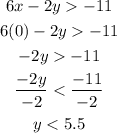
We have the point: (0, 5.5)

When y=0

`\begin{gathered} 6x-2y>-11 \\ 6x>-11 \\ x>(-11)/(6) \\ x>-1.83 \end{gathered}`

We have the point: (-1.83, 0)

We plot the points (0, 5.5) and (-1.83,0).

The points are joined using a broken line because of the inequality sign: >

The graph is shown below:

To determine which side is required, we use the origin test.

When x=0 and y=0

`\begin{gathered} 6x-2y>-11 \\ 0>-11\text{ (TRUE)} \end{gathered}`

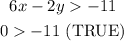
This means the side of the line containing (0,0) is where we need. (Shaded above)