Leave the variable in each equation in one side of the equation to find if it has a solution:
a.

As 4 is not equal to 5, the equation has no solution.
b.
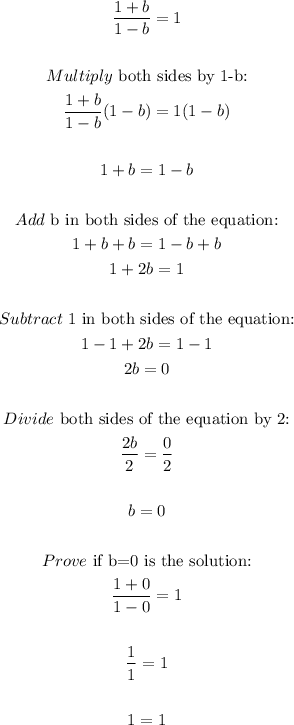
The solution for the equation is b=0
c.
![\begin{gathered} (c-5)/(5-c)=1 \\ \\ Multiply\text{ both sides by \lparen5-c\rparen:} \\ (c-5)/(5-c)(5-c)=1(5-c) \\ \\ c-5=5-c \\ \\ Add\text{ c in both sides of the equation:} \\ c+c-5=5-c+c \\ 2c-5=5 \\ \\ Add\text{ 5 in both sides of the equation:} \\ 2c-5+5=5+5 \\ 2c=10 \\ \\ Divide\text{ both sides by 2:} \\ (2c)/(2)=(10)/(2) \\ \\ c=5 \\ \\ Prove\text{ if c=5 is a solution:} \\ (5-5)/(5-5)=1 \\ \\ (0)/(0)=1 \\ \\ (0)/(0)\text{ is undefined} \end{gathered}]()
As the possible solution (c=5) makes the expression has a undefined part (0/0) it is not a solution.
The equation has no solution