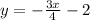Answer:
The equation of the line is
Explanation:
Equation of a line:
The equation of a line has the following format:
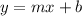
In which m is the slope and b is the y-intercept.
Perpendicular lines:
When two lines are perpendicular, the multiplication of their slopes is -1.
Perpendicular to the line 4x-3y=18
First, we place this line into the format, to find the slope:
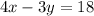
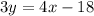

This line has slope 4/3. So, for the perpendicular line, the slope will be of m as such:

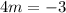
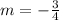
So the desired line will have an equation in the following format:
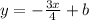
Passes through the point (8,-8)
We use this to find b. This point means that when
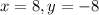 . So
. So
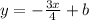
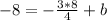

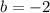
So