Let's compare the given equation with the notable product below:
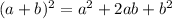
Since the first term is 4x², we have a = 2x.
Then, the second term is 8x, so since a = 2x, we have b = 2, this way 2ab = 8x
Now, since b = 2, we have b² = 4.
The constant term is just 1, so we need to add 3 units:
![\begin{gathered} 4x^2+8x+1+3-3=0 \\ 4x^2_{}+8x+4-3=0 \\ (2x+2)^2=3 \\ 2x+2=\pm\sqrt[]{3} \\ 2x=-2\pm\sqrt[]{3} \\ x=\frac{-2\pm\sqrt[]{3}}{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/9vjvodylmg1impytkvwx2d4dh7xavih387.png)
Therefore the correct option is the third one.