Answer:
D) y = 3
Step-by-step explanation:
We were given that:
AC = 3y + 13
BD = 28 - 2y
From the diagram, for the figure to be an isosceles trapezoid, that would imply that diagonals AC & BD are congruent
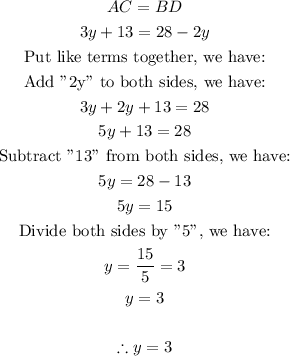
The value of "Y" that makes the diagram an isosceles trapezoid is: y = 3
Therefore, the correct option is D