Okay, here we have this:
Considering the provided information, we are going to calculate the requested time, so we obtain the following:
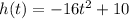
And he caught them at a height of 4 feet, so we'll substitute and solve for t:
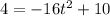
Solving:
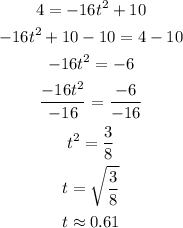
Finally we obtain that the time where approximately 0.61.