Question

Is number three connected to number two? I did number two and have the answer for it but I don’t know if number three separate or not can you please help me with a number three

Answer 1

The question requires an understanding of what complementary angles are.

Definition:

Complementary angles are angles that add up to 90 degrees.

This means that Angles A and B add up to 90 degrees.

Given that:

`\begin{gathered} A=x \\ B=3x+6 \end{gathered}`

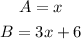
It means that we can write the addition of A and B equalling 90 degrees.

`\begin{gathered} A+B=90^0 \\ x+3x+6=90 \end{gathered}`

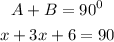
Now, let us solve for x. Once we have x, we can find angles A and B.

`\begin{gathered} x+3x+6=90 \\ 4x+6=90 \\ \text{subtract 6 from both sides} \\ 4x=90-6 \\ 4x=84 \\ \text{divide both sides by 4} \\ (4x)/(4)=(84)/(4)=(21*4)/(4)\text{ ( 4 crosses out)} \\ \\ \therefore x=21 \end{gathered}`

Now that we have the value of x, we can find A and B. Let us do that now:

`\begin{gathered} A=x \\ \therefore A=21 \\ \\ B=3x+6 \\ B=3(21)+6 \\ B=63+6=69^0 \\ \\ \therefore A=21,B=69 \end{gathered}`

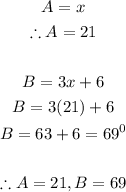
Therefore, the final answer is:

A = 21, B= 69