Given:
An exponential function

for a graph that passes through (2, 1) and (3, 8).
To know:
Find exponential function.
Step-by-step explanation:
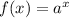
Solution:
We will use given values (2, 1) and (3, 8) in function
For point (2, 1)
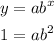
At (3, 8)
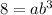
So,
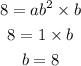
Now, from value b ,we can find a,
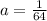
So,
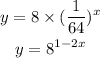
Hence, this is the function.