ANSWER
The larger angle is 71° and the smaller angle is 19°.
Step-by-step explanation
Let the first angle be x.
Let the second angle be y.
The two angles add up to 90 degrees. This implies that:
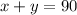
The difference between the two angles is 52. This implies that:
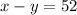
Now, we have a system of simultaneous equations:
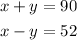
To solve by elimination, add the two equations to eliminate y:
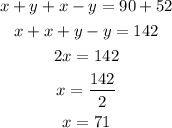
To find the value of y, substitute the value of x into the first equation:

Hence, the larger angle is 71° and the smaller angle is 19°.