Question

A bank teller has a total of 35 bills, made up of $5 bills and $10 bills. If the total value of the money is $200, how many bills of each denomination does she have?

Answer 1

The bank teller has 30 $5-bills and 5 $10-bills.

Step-by-step explanation:

• Let the number of $5 bills = x

,

• Let the number of $10 bills = y

The bank teller has a total of 35 bills.

`\implies x+y=35\cdots(1)`

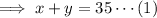
The total value of the money is $200.

`\begin{gathered} \text{The value of x \$5 bills = 5x} \\ \text{The value of y \$10 bills = 10y} \\ \implies5x+10y=200\cdots(2) \end{gathered}`

We can divide equation (2) all through by 5:

`\begin{gathered} (5x)/(5)+(10y)/(5)=(200)/(5) \\ x+2y=40\cdots(3) \end{gathered}`

Solve equations (1) and (3) simultaneously:

`\begin{gathered} x+2y=40\cdots(3) \\ x+y=35\cdots(1) \\ \text{Subtract } \\ y=5 \end{gathered}`

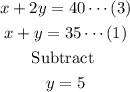
Solve for x:

`\begin{gathered} x+y=35 \\ x+5=35 \\ x=35-5 \\ x=30 \end{gathered}`

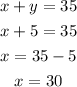
x=30 and y=5

Thus, the bank teller has 30 $5-bills and 5 $10-bills.