Question

A gas occupies 60.0 mL at 20°C and 770 mm Hg. What volume will it occupy at 40°C and 735 mm Hg?115 mL67.1 mL58.8 mL61.2 mL

Answer 1

The final volume of the gas is 67.1 mL

Step-by-step explanation

Given that;

The initial volume of the gas is 60.0mL

The initial temperature of the gas is 20 degrees Celcius

The initial pressure of the gas is 770 mmHg

The final temperature of the gas is 40 degrees Celcius

The final pressure of the gas is 735 mmHg

Follow the steps below to find the final volume of the gas

Step 1; Write the general gas law equation

`\text{ }\frac{\text{ P1 V1}}{\text{ T1}}\text{ }=\text{ }\frac{\text{ P2 V2}}{\text{ T2}}`

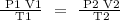
Step 2; Convert the temperature to degrees kelvin

`\begin{gathered} \text{ T K = t + 273.15} \\ \text{ for t1 = 20}\degree C \\ \text{ T = 20 + 273.15} \\ \text{ T = 293.15K} \\ \\ \text{ For t2 = 40}\degree C \\ \text{ T = 40 + 273.15} \\ \text{ T = 313.15K} \end{gathered}`

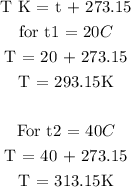
Step 3; Substitute the given data into the formula

`\begin{gathered} \text{ }\frac{770\text{ }*\text{ 60}}{293.15}=\text{ }\frac{\text{ 735}*\text{ V2}}{313.15} \\ \text{ Cross multiply} \\ \text{ 770}*\text{ 60 }*\text{ 313.15 = 735 }*\text{ V2 }*\text{ 293.15} \\ 14,467,530\text{ = V2 }*215,465.25 \\ \text{ Isolate V2} \\ \text{ V2 = }(14,467,530)/(215,465.25) \\ \text{ V2 = 67.1mL} \end{gathered}`

Therefore, the final volume of the gas is 67.1 mL