ANSWER
5.9 inches
Step-by-step explanation
We have to find the area of the horizontal cross-section of the cylinder.
The horizontal cross-section of a cylinder is the shape that you get when you slice through a cylinder horizontally.
That is:
Therefore, we have to find the area of the circular shape.
The area of a circle is given as:
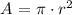
where r = radius
From the question, the diameter is 2.75 inches. Since radius is half of the diameter, the radius is:
r = d / 2 = 2.75 / 2
r = 1.375 inches
Therefore, the cross-sectional area is:
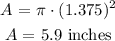
That is the answer.