Total number of transistors (n) = 24.
Several defective transistors (r) = 4.
The total number of ways of selecting four transistors is,
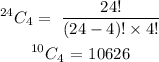
(a) The probability that exactly two are defective is calculated as,
![P(Exactly\text{ 2 are defective) = }\frac{Ways\text{ of selecting defective}* Ways\text{ of selecting non defective}}{Total\text{ number of ways of selecting }4\text{ transistors}}]()
Therefore,
![\begin{gathered} P(Exactly\text{ 2 are defective) = }(^4C_2*^(20)C_2)/(^(24)C_4) \\ P(Exactly\text{ 2 are defective) = }(6*190)/(10626) \\ P(Exactly\text{ 2 are defective) = }(1140)/(10626) \\ P(Exactly\text{ 2 are defective) = 0.1073} \end{gathered}]()
(b) The probability that none of them are defective is calculated as,
![\begin{gathered} P(\text{None of them are defective) = }\frac{No\text{. of ways of selecting }non\text{ defectives}}{\text{Total number of ways}} \\ P(\text{None of them are defective) = }\frac{^(20)C_{^{}4}}{^(24)C_4} \\ P(\text{None of them are defective) = }(4845)/(10626) \\ P(\text{None of them are defective) = }0.4560 \end{gathered}]()
(c) The probability that all of them are defective is calculated as,
![\begin{gathered} P(All\text{ of them are defective) = }(^4C_4)/(^(24)C_4) \\ P(All\text{ of them are defective) = }(1)/(10626) \\ P(All\text{ of them are defective) = }0.00009 \end{gathered}]()
(d) The probability that atleast one of them is defective is calculated as,
![\begin{gathered} P(atleast\text{ one is defective) = }1\text{ - P(no defective)} \\ P(atleast\text{ one is defective) = }1-\text{ 0.4560} \\ P(atleast\text{ one is defective) = }0.5440 \end{gathered}]()