Answer:
Length = 12 miles
Width = 6 miles
Explanation:
Set up our two equations:
L * W = 72
W = L - 6
So, let's plug W in to find our values:
(Remember: W = L - 6)
L * (L - 6) = 72
Simplify:
L^2 - 6L = 72
L^2 - 6L - 72 = 0
Let's use the quadratic formula (a = 1, b = -6, c = -72)
Quadratic formula:
x = -b ±

______________
2a
Plug in our values:
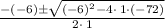
Solve for the square root value:
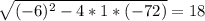
Set up the equation with simplified values:
L, W =
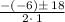
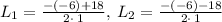
Solve for L1:
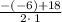 = 12
= 12
Solve for L2:
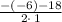 = 6
= 6
Since the width is 6 miles less than the length, the width is 6 miles, and the length is 12 miles.
Plug this in to make sure: Does 6 * 12 = 72? Yes. Is 12 6 more than 6? Yes. We have our answers
Length = 12 miles
Width = 6 miles