Question

A complex electronic system is built with a certain number of backup components in its subsystems. One subsystem has four identical components, each with a probability of 0.2 of failing in less than 1000 hours. The subsystem will operate if any two of the four components are operating. Assume that the components act independently.a) Find the probability that exactly two of the four components last longer than 1000 hours.b) Find the probability that the subsystem operates longer than 1000 hours.

Answer 1

a) 0.1536 = 15.36% probability that exactly two of the four components last longer than 1000 hours.

b) 0.9728 = 97.28% probability that the subsystem operates longer than 1000 hours.

Explanation:

For each component, there are only two possible outcomes. Either they fail in less than 1000 hours, or they do not. The probability of a component failing in less than 1000 hours is independent of any other component. This means that we use the binomial probability distribution to solve this question.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

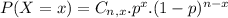
In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

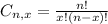
And p is the probability of X happening.

One subsystem has four identical components, each with a probability of 0.2 of failing in less than 1000 hours.

Four components means that
`n = 4`

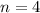
Probability of 0.2 of failling means that 1 - 0.2 = 0.8 probability of not failling, so
`p = 0.8`

a) Find the probability that exactly two of the four components last longer than 1000 hours.

This is
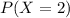`P(X = 2)`. So

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 2) = C_(4,2).(0.8)^(2).(0.2)^(2) = 0.1536`

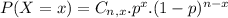
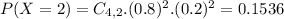
0.1536 = 15.36% probability that exactly two of the four components last longer than 1000 hours.

b) Find the probability that the subsystem operates longer than 1000 hours.

It will operate if at least two compoents last longer than 1000 hours. So

`P(X \geq 2) = P(X = 2) + P(X = 3) + P(X = 4)`

In which

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 2) = C_(4,2).(0.8)^(2).(0.2)^(2) = 0.1536`

`P(X = 3) = C_(4,3).(0.8)^(3).(0.2)^(1) = 0.4096`

`P(X = 4) = C_(4,4).(0.8)^(4).(0.2)^(0) = 0.4096`

`P(X \geq 2) = P(X = 2) + P(X = 3) + P(X = 4) = 0.1536 + 0.4096 + 0.4096 = 0.9728`

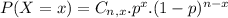
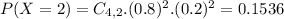
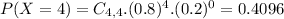
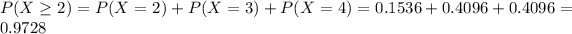
0.9728 = 97.28% probability that the subsystem operates longer than 1000 hours.