To answer this question, we need to find the area of the rectangle with sides 14 cm and 9 cm, and, then, we need to subtract from this area, the area of the semicircle with radius = 7 cm (that is, 14 cm /2 = 7 cm).
Therefore, we have:
1. Find the area of the rectangle:
![A=14\operatorname{cm}\cdot9\operatorname{cm}=126\operatorname{cm}^2]()
2. Find the area of the semicircle:
The area of a circle is given by:
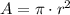
Therefore, for a semicircle, we have:
![a=(\pi\cdot r^2)/(2)\Rightarrow a=(\pi\cdot7^2)/(2)\Rightarrow a=76.9690200129\operatorname{cm}^2]()
Then, we need to subtract this value from the obtained in calculating the area of the rectangle:
![126\operatorname{cm}-76.96902\operatorname{cm}=49.03098\operatorname{cm}^2]()
From the question, the answer is option D, 49.07 cm squared.