Question

9. A zoo has two water tanks that are leaking. One tank contains 100 gal of water and is leaking at a constant rate of 4 gal/h. The second tank contains 60 gal of water and is leaking at a constant rate of 2 gal/h. When will the tanks have the same amount of water?

Answer 1

we can write an equation for each statement

One tank contains 100 gal of water and is leaking at a constant rate of 4 gal/h

`y=100-4h`

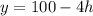
where y are the total gallons and H the hours elapsed

he second tank contains 60 gal of water and is leaking at a constant rate of 2 gal/h.

`y=60-2h`

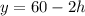
where y are the total gallons and H the hours elapsed

When will the tanks have the same amount of water?​

we must replace one equation in the other to find the time that its measure is equal,so

`100-4h=60-2h`

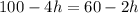
now solve h

`\begin{gathered} 100-60=4h-2h \\ 40=2h \\ (40)/(2)=h \\ \\ h=20 \end{gathered}`

the two tanks will have the same volume when 20 hours have passed