ANSWER
The other two zeros are
• 3 + 2i
,
• 3 - 2i
Step-by-step explanation
If the zeros of a polynomial are x1, x2, x3... the polinomial function can be written in a factored form,
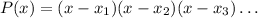
Hence, if we know that one of the zeros of f(x) is -4, that means that (x + 4) is a factor. Thus, we can divide the polynomial by that factor,
So f(x) is,
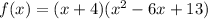
To find the other two zeros now we just have to find the zeros of the second factor (x² - 6x + 13), which is much easier because we can simply use the quadratic formula,
![\begin{gathered} ax^2+bx+c=0 \\ x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/uuterq6bz1kwr2mb9jy58c523r5v4v644y.png)
In this case a = 1, b = -6 and c = 13,
![x=\frac{6\pm\sqrt[]{6^2-4\cdot1\cdot13}}{2\cdot1}](https://img.qammunity.org/2023/formulas/mathematics/high-school/8r10anjtuut3e2whnc9xsso5cng2m8z1fz.png)
![x=\frac{6\pm\sqrt[]{36-52}}{2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/jrf9t8anb4sx692h0wybh90bwh3zfleifx.png)
![x=\frac{6\pm\sqrt[]{-16}}{2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/fzx80kpqgngeypgbglt7zoyw6n4axfuy0y.png)
Note that the number under the radical is negative. Therefore the other two zeros are not real - in other words, in the real numbers set this function has only one zero: -4.
In the complex number set we know that i² = -1, so we can replace the minus sign under the radical by i²,
![x=\frac{6\pm\sqrt[]{16i^2}}{2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/xrz5c8j59sgusezaylvpj0iiejws1gs1vt.png)
And solve the square root,

We can distribute the denominator into the sum/subtraction,
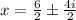
And we get that the other two zeros are,
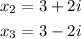
This agrees with the complex conjugate root theorem, which states that