Question

Solve each system by substitution.y - 4x = 3 2x - 3y = 211. Rewrite the first equation in terms of . y=2. Substitute the value of into the second equation. 2x - 3 = 213. Solve for X .4. Substitute the value of in one of the original equations. Solve for y .5. Write your answer as an ordered pair (x,y) .Solution: ( , )

Answer 1

We have to solve this system by substitution.

This method let us define a varaible in function of the other (or others, if they are more than two) and then replace it in the other equations by this relation.

The system is:

`\begin{gathered} y-4x=3 \\ 2x-3y=21 \end{gathered}`

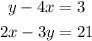
1. From the first equation, we can easily define y in function as x:

`\begin{gathered} y-4x=3 \\ y=4x+3 \end{gathered}`

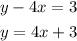
2. Now, we substitute y in the second equation:

`\begin{gathered} 2x-3y=21 \\ 2x-3(4x+3)=21 \end{gathered}`

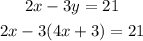
3. We got an equation that is expressed only in function of x, so we can solve it as:

`\begin{gathered} 2x-3(4x+3)=21 \\ 2x-3\cdot4x-3\cdot3=21 \\ 2x-12x-9=21 \\ -10x=21+9 \\ -10x=30 \\ x=(30)/(-10) \\ x=-3 \end{gathered}`

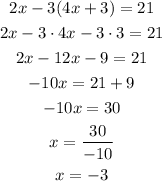
4. If we replace x in the equation for y, we get:

`\begin{gathered} y=4x+3 \\ y=4(-3)+3 \\ y=-12+3 \\ y=-9 \end{gathered}`

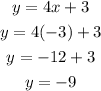
5. Then, we can write the answer a s a pair (x,y) = (-3,-9)