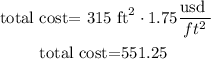$551.25
Step-by-step explanation
Step 1
convert the mixed numbers in fractions
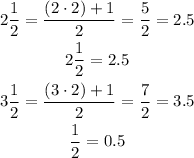
Step 2
find the factor of scale
remind 1 feet =12 inches

it means, if you measure a inch in the drawing it corresponds to 72 inches in the real the room
Step 3
find the real measures

Step 4

Step 5
find the area of the room

Step 6
finally, to obtain the total cost of the carpet, multiply the number of square feet by the cost per square foot of the carpet