Question

5. Assume that the sales of a certain automobile parts company are approximated by a linear function. Suppose that sales were $200,000 in 1981 and $1,000,000 in 1988. Let x = 0 represent 1981 and x = 7 represent 1988. (a) Find the equation giving the company's yearly sales. (b) Find the approximate sales in 1983. (c) Estimate sales in 1999.

Answer 1

a)

The equation of the line in the slope-intercept form has the next form

`y=mx+b`

where m is the slope and b is the y-intercept

So first we need to calculate the slope, the slope is given by the next formula

`m=(y_2-y_1)/(x_2-x_1)`

where (x1,y1) and (x2,y2) are points where the line passes through.

In our case

(0,200000)

(7,1000000)

We substitute the data

`m=(1,000,000-200,000)/(7-0)=(800000)/(7)`

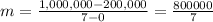
Then we need to calculate the y-intercept that is b

x=0 and y=200000

`200000=(800000)/(7)(0)+b`

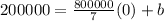
We isolate the b

`b=200000`

The linear equation is

`y=(800000)/(7)x+200000`

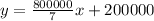
b)

If x=0 represents 1981, therefore x=2 represents 1983

We substitute in the equation above x=2

`y=(800000)/(7)(2)+200000=(3000000)/(7)=428571.43`

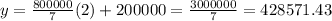
The approximate sales in 1983 are $428571.43

c)

if x=0 represents 1981, therefore x=18 represents 1999

`y=(800000)/(7)(18)+200000=2257142.86`

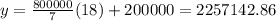
The estimated sales in 1999 are $2257142.86