Given data:
Initial velocity of the ball;
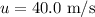
Launch angle;
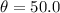
Distance between the launch site and the hole;
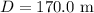
The range of the projectile is given as,

Here, g is the acceleration due to gravity.
Substituting all known values,
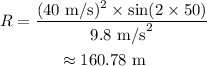
The distance between the hole and landing site is given as,
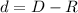
Substituting all known values,
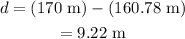
Therefore, the ball will land 9.22 m away from the hole.