Answer:
1.- We are given that:
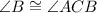
therefore:
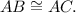
2.- Recall that a condition that must be satisfied for three lengths to be the sides of a triangle is that the sum of any pair of lengths is greater than the other length, therefore:

3.- Substituting AC for AB, using the congruence statement in point number 2, we get:
