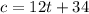Question

During the first year of opening a law firm , a lawyer served 46 clients .in the second year, his number grew to 58 . If the linear trend continue, write an equation that gives the number of clients (c) the lawyer will have have (t) years after beginning the firm

Answer 1

The equation of a line with slope m and y-intercept b in slope-intercept form is:

`y=mx+b`

The slope represents the rate of change of the variable y with respect to the variable x, and the y-intercept represents the initial value of y when x=0.

In this case, let c represent the number of clients as a function of time, and let t represent time in years.

Then, c=46 when t=1 and c=58 when t=2.

Use the slope formula to find the slope of the line that passes through the points (1,46) and (2,58) in a c vs t graph:

`\begin{gathered} m=(c_2-c_1)/(t_2-t_1) \\ =(58-46)/(2-1) \\ =(12)/(1) \\ =12 \end{gathered}`

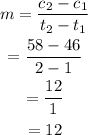
Replace 12 for the slope and substitute a pair of corresponding values of c and t into the equation to find the initial value. For instance, substitute t=1 and c=46:

`\begin{gathered} c=12t+b \\ \Rightarrow46=12(1)+b \\ \Rightarrow46-12=b \\ \Rightarrow34=b \\ \therefore b=34 \end{gathered}`

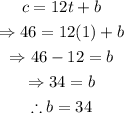
To find the equation that gives the number of clients the lawyer will have as a function of time, replace 12 for the slope and 34 for the initial value:

`c=12t+34`

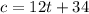
We can verify that we obtain the correct values for c when t=1 and t=2:

`\begin{gathered} c_1=12(1)+34=12+34=46 \\ c_2=12(2)+34=24+34=58 \end{gathered}`

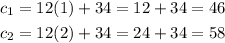
Therefore, the equation that gives the number of clients (c) the lawyer will have (t) years after beginning the firm, is:

`c=12t+34`