Given data:
* The linear speed of the cyclist is v = 19 miles per hour.
* The diameter of the circular motion is d = 30 inches.
Solution:
The radius of the circular motion is,
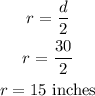
The radius of the circular path in terms of feet is,
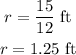
The linear velocity of the cyclist in terms of feet per minute is,

The angular speed of the cyclist is,
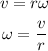
Substituting the known values,
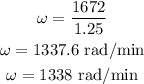
Thus, the angular speed of the cyclist is 1338 radian per minute.
(b). The number of revolutions in one minute is,
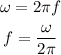
Substituting the known values,
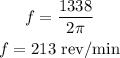
Thus, the number of revolutions per minute is 213 rev/min.