The expression for equation of a straight line is given as

An expression for m, the slope is given as
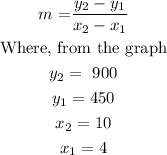
Therefore, the slope is given as
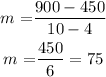
The slope = 75
The slope represents the hourly rate charged
The equation for C , is C = 75t + c
From the graph c = 150
Therefore, the required final equation for C is
C = 75T + 150
C represents the total cost of all services the electrician provided
In 1 hours, his total cost will be
C = 75(1) + 150
C = $225