Question

I need help with this practice problem In the picture is MY attempted answer

Answer 1

To determine the value of sin (θ + β), we can apply the trigonometric identity:

`\sin (\theta+\beta)=\sin \theta\cos \beta+\cos \theta\sin \beta`

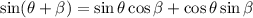
Since we already have the value for cosθ, let's find out sin θ.

Based on trigonometric identity,

`\sin \theta=(y)/(r);\cos \theta=(x)/(r)`

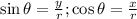
Based on the given value of cosθ, x = -√2 while r = 3. To determine the value of y, let's apply the Pythagorean Theorem.

`\begin{gathered} y=\sqrt[]{r^2-x^2} \\ y=\sqrt[]{3^2-(-\sqrt[]{2})^2} \\ y=\sqrt[]{9-2} \\ y=\sqrt[]{7} \end{gathered}`

Since the given interval is between π and 3π/2 which is in Quadrant 3, y = -√7. Hence, the value of sin θ is:

`\sin \theta=-\frac{\sqrt[]{7}}{3}`

The next thing that we shall solve is sin β and cos β. We can use the given tangent function to determine this.

`\begin{gathered} \text{tan}\beta=(y)/(x)=(4)/(3) \\ \text{Solve for r.} \\ r=\sqrt[]{x^2+y^2}=\sqrt[]{3^2+4^2}=\sqrt[]{9+16}=\sqrt[]{25}=5 \end{gathered}`

Given the interval for beta, the angle is found in Quadrant 1. So, the values of sin β and cos β are:

`\begin{gathered} \sin \beta=(y)/(r)=(4)/(5) \\ \cos \beta=(x)/(r)=(3)/(5) \end{gathered}`

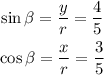
Now that we have the values for sin θ = -√7/3, cos β = 3/5, cos θ = -√2/3, and sin β = 4/5, let's plugged them into the first trigonometric identity we mentioned above.

`\begin{gathered} \sin (\theta+\beta)=\sin \theta\cos \beta+\cos \theta\sin \beta \\ \sin (\theta+\beta)=(-\frac{\sqrt[]{7}}{3})((3)/(5))+(-\frac{\sqrt[]{2}}{3})((4)/(5)) \end{gathered}`

Then, simplify.

`\sin (\theta+\beta)=-\frac{\sqrt[]{7}}{5}-\frac{4\sqrt[]{2}}{15}`

The final answer is shown above.