We have the followed expression for a giving line:
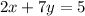
Isolating the Y we find the expression:

We know that if a line with expression y=mx+b is perpendicular to the line that the question gave to us, the product of the slopes of the two lines must be:
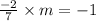
With this last expression, we can find the slope for the line perpendicular to our first line:
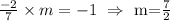
So, our slope is 7/2