Answer:
Step-by-step explanation:
Here, we want to get the plot of the parabola
We start by getting its vertex
We have that as follows:
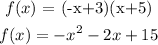
We have the vertex form as follows:
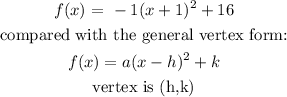
The vertex here is thus (-1,16)
To get the other point, we can equate the values in the brackets to zero and solve for x
We have that as:
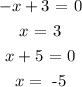
The other points are thus (3,0) and (-5,0)
Thus, we can join (-1,16) with either (3,0) and (5,0) as shown below: