Question

Solve this problem using system of linear equationsA chef is going to use a mixture of two brands of Italian dressing. The first brand contains 7% vinegar, and the second brand contains 12% vinegar. The chef wants to make 210 milliliters of a dressing that is 11% vinegar. How much of each brand should she use? First brand=( ) MillilitersSecond brand=( )Milliliters

Answer 1

Let's call x the vinegar.

• The first brand contains 7% vinegar, this can be expressed as ,0.07x,.

,

• The second brand contains 12% vinegar, this can be expressed as ,(210-x)*0.12, because the total amount is 210 mL.

,

• The chef wants to make 210mL which is 11% vinegar, this is going to be the other side of the equation.

Let's express the equation.

`0.07x+(210-x)\cdot0.12=0.11\cdot210`

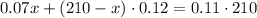
Now we solve for x.

`\begin{gathered} 0.07x+25.2-0.12x=23.1 \\ -0.05x=23.1-25.2 \\ x=(-2.1)/(-0.05) \\ x=42 \end{gathered}`

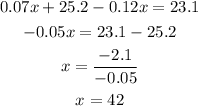
Therefore, the chef needs 42 mL for the first brand and 168 mL for the second one.