Question

Is the degree of the function even, odd or neither?

Answer 1

the degree of the function is neither

Step-by-step explanation:

A function is even if for values of x:

`f\mleft(x\mright)=f\mleft(-x\mright)`

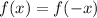
A function is odd if for the values of x:

`f(x)\text{ = }-f(-x)`

We can test few x values on the graph to determine if it is even or odd:

`\begin{gathered} \text{for even: }f(x)=f(-x) \\ \text{when x = 1} \\ f(1)\text{ = 0} \\ \text{-x = -1} \\ \text{when x = -1} \\ f(-1)\text{ = }-8 \\ 0\text{ }\\e\text{ -8} \\ \\ f(1)\text{ }\\e\text{ f(-1)} \\ H\text{ence, it is not even} \end{gathered}`
`\begin{gathered} \text{for odd: }f(x)\text{ = }-f(-x) \\ \text{when x = 2} \\ f(2)\text{ = }12 \\ -x\text{ = -2} \\ \text{when x = -2} \\ f(-2)\text{ = -20} \\ -f(-x)\text{ = -f(-2) = -}(-20) \\ -f(-x)\text{ = 20} \\ 12\text{ }\\e\text{ 20} \\ \\ f(2)\text{ }\\e\text{ }-f(-2) \\ \text{Hence, it is not odd} \end{gathered}`

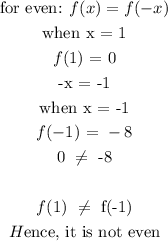
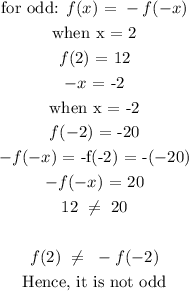
Since the function is not even nor odd, then the degree of the function is neither