ANSWER
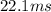
Step-by-step explanation
Mass of the pig, m = 300 kg
Length of the hill = 200 m
Vertical drop of the hill, h = 25 m
To find the speed of the pig at the bottom of the hill, we apply the conservation of energy. At the start of the drop, the pig only has potential energy while at the end, it only has kinetic energy.
Hence, apply the law of conservation of energy:

where g = acceleration due to gravity
Substitute the given values into the equation and solve for speed, v:
![\begin{gathered} v^2=2gh \\ \Rightarrow v=\sqrt[]{2gh} \\ v=\sqrt[]{2\cdot9.8\cdot25} \\ v=\sqrt[]{490} \\ v=22.1m\/s \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/rh1n2lddapkjtl0u9jzsax68aqfyycxn35.png)
That is the speed of the pig at the bottom of the hill.