Given
Part 1
Jane walked for 3 km at a constant speed of x km/hr
speed x km/h and
distance is 3km

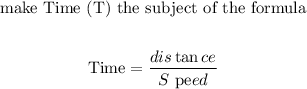
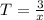
She then jogged for a further x km at a constant speed of 8 km/hr
Therefore, Distance is X km and
Speed is 8km/hr

The total time is;

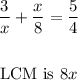
Multiply all through by the LCM
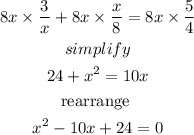
Part 2
We have to solve the quadratic equation

Recall From the question
if her total distance was less than 8 km.
![\begin{gathered} 3\operatorname{km}\text{ +6km = 9km} \\ It\text{ is greater than 8km, so it not 6} \\ \\ \text{Then} \\ 4\operatorname{km}\text{ +3km =7km} \\ \text{Then, it is 4} \end{gathered}]()
The speed X is 4km/h