Question

Find the sum of the first 85 terms of the sequence –3, –1, 1, 3, …Select one:a.6804b.6887c.6885d.6881

Answer 1

Given:

The sequence is: -3, -1, 1, 3,..

Required: The sum of the first 85 terms

The sequence is a an arithmetic progression where:

first term (a) = -3

common difference (d) = 2

The formula for the sum of the first n terms of an arithmetic progression is:

`S_n=\text{ }(n)/(2)[2a\text{ + \lparen n-1\rparen d\rbrack}`

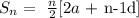
For this problem, n = 85

Substituting the given values:

`\begin{gathered} S_(85)\text{ = }(85)/(2)[2*-3\text{ + \lparen85-1\rparen}*2] \\ =\text{ 6885} \end{gathered}`

Answer: Option C