Given:
point =(4,4) slope(m)=-4/3
x₁=4 y=4
We find the intercept
substitute into y=mx + b
4=4(-4/3)+ b
4 = -16 /3 + b
4+16/3 = b
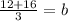
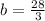
The equation can be form by substituting the value of m and b into y=mx+b
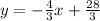
To be able to sketch the graph, we need two points, the x and y-intercept.
To find the y-interpet, substitute x=0 into the equation
y = 28/3
Hence, the y-intercept is (0, 9.3)
To find the x-intercept, put y=0 and solve for x
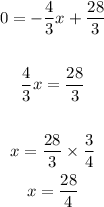
The x-intercept is (7,0)
So we can now sketch the graph