We are given a right triangle and we are asked to determine the angle B given the opposite and adjacent sides. To do that we will use the function tangent since this is defined as:
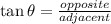
Substituting the values we get:
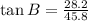
Now, we use the inverse function of the tangent:
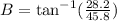
Solving the operations:
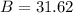
Therefore, angle B is 31.62°.