Question

Linear Algebra "If you buy 9 articles of clothing, the end cost will be 264. 1 shirt costs $24, and 1 pant $36, solve these using systems of equations"I do not know what they are asking or HOW to get the answer with systems of equations (a requirement) Please help I am so upset

Answer 1

Let x be the number of shirts and y be the number of pants, since the total number of articles must be 9, we can write

`x+y=9\ldots(i)`

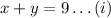
Additionally, we know that the total cost must be $264 and 1 shirt cost $24 and 1 pant $36, then we can write

`24x+36y=264\ldots(ii)`

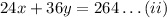
Then, we have the following system of equations:

`\begin{gathered} x+y=9 \\ 24x+36y=264 \end{gathered}`

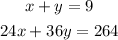
Solving by elimination method.

By multiplying the first equation by -24, we can obtain an equivalent system of equations:

`\begin{gathered} -24x-24y=-216 \\ 24x+36y=264 \end{gathered}`

Then, by adding both equations, we have

`0+12y=48`

Then, we get

`\begin{gathered} 12y=48 \\ y=(48)/(12) \\ y=4 \end{gathered}`

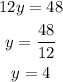
Now, in order to find x, we can substitute this result into equation (i). It yields,

`x+4=9`

which gives

`\begin{gathered} x=9-4 \\ x=5 \end{gathered}`

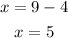
Therefore, the answer is 5 shirts and 4 pants.