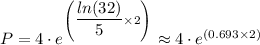Answer:
The function that can be used to describe the number (n) of bacteria after 2 minutes is;
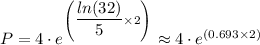
Explanation:
The data in the table are presented as follows;
Number of bacteria; 4, 128, 4,096, 131,072
Number of minutes from initial state; 0, 5, 10, 15
The general equation for population growth is presented as follows;
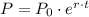
Where;
P = The population after 't' minutes
P₀ = The initial population
r = The population growth rate
t = The time taken for the growth in population numbers
At t = minutes. we have;

∴ P₀ = 4
At t = 5, we have;
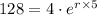
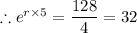
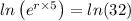
∴ r × 5 = ㏑(32)
r = ln(32)/5 ≈ 0.693
The number (n) of bacteria after 2 minutes is therefore;