Question

Instructions: Calculate the discriminant, then state the number and type of solutions.

Answer 1

Given:

`-9x^2-7x-2=0`

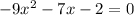
To find:

The discriminant

Step-by-step explanation:

Using the discriminant formula,

`D=b^2-4ac`

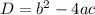
Here,

`\begin{gathered} a=-9 \\ b=-7 \\ c=-2 \end{gathered}`

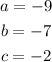
The discriminant value becomes,

`\begin{gathered} D=(-7)^2-4(-9)(-2) \\ =49-72 \\ =-23 \\ <0 \end{gathered}`

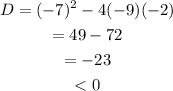
Therefore, it has imaginary solutions.

Ans there are two solutions.

Final answer:

• There are imaginary solutions to the quadratic equation with this discriminant.

,

• There are 2 solutions.