Question

The mean Exam score on the SOC 15 Exam 2 is 84.28 with a standard deviation of 6.71.You score a 92 on the exam. What percentage of students scored higher than you on the exam?

Answer 1

First, find the z-score of 92 using the formula

`\begin{gathered} z=(x-\mu)/(\sigma) \\ \\ \text{Substitute the following} \\ x=92 \\ \mu=84.28 \\ \sigma=6.71 \end{gathered}`
`\begin{gathered} z=(x-\mu)/(\sigma) \\ z=(92-84.28)/(6.71) \\ z=(7.72)/(6.71) \\ z=1.15 \end{gathered}`

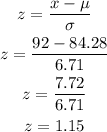
Look for the value of z-score in the z-table

Since we are looking for percentage of higher than 92, subtract 0.87493 from 1

`\begin{gathered} P(Z>1.15)=1-0.87493 \\ P(Z>1.15)=0.12507 \end{gathered}`

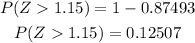
Multiply the result by 100% to get the percentage.

`0.12507\cdot100\%=12.507\%`

Therefore, the percentage of students who scored higher than you on the exam is 12.507%.