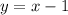Question

What is the equation of the line that is parallel to the line y = x + 4 and passes through the point (6, 5)?y = x + 3y = x + 7y = 3x – 13y = 3x + 5

Answer 1

You have to find a line that is parallel to y=x+4 and passes through the point (6,5)

Parallel lines always have the same slope.

The slope of the line is the coefficient that multiplies the x term, in this case, there is no number multiplying "x" but that does not mean that the line has no slope, turns out that the line has a slope equal to "1" when the variables (letters in an equation) are "alone" the coefficient is 1

So for both lines, the slope is

`m=1`

Using the slope and the known point of the line, you can determine the equation of said line by using the "point-slope" form

`y-y_1=m(x-x_1)`

Where

(x₁,y₁) are the coordinates of a point of the line

m is the slope

Replace the coordinates of the point and the slope in the formula

`y-5=1(x-6)`

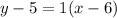
Next is to solve for y to express it in slope-intercept form

`\begin{gathered} y-5=1\cdot x-1\cdot6 \\ y-5=x-6 \\ y-5+5=x-6+5 \\ y=x-1 \end{gathered}`

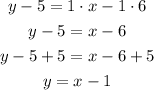
The equation for a line parallel to y=x+4 that passes through point (6,5) is

`y=x-1`