Question

Find the equation of the line thatis parallel to y = 2x + 5 and 6contains the point (2,-4).y = [? ]X + [ ]Enter

Answer 1

The general equation of a line in slope-intercept form is given by:

`y=mx+c`

Given the equation of a line to be

`y=2x+5`

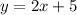
Upon comparing the equation to the general line equation, then

`\begin{gathered} m=2 \\ c=5 \end{gathered}`

Next, to find the equation of the line parallel to the given line

we will use the formula

`y-y_1=m(x-x_1)`

where

`\begin{gathered} m=2 \\ x_1=2 \\ y_1=-4 \end{gathered}`

`\begin{gathered} y-(-4)=2(x-2) \\ y+4=2x-4 \\ y=2x-4-4 \\ y=2x-8 \end{gathered}`

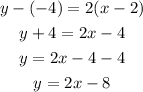
The equation of the line is y=2x -8