Question

A uniform-density 7 kg disk of radius 0.20 m is mounted on a nearly frictionless axle. Initially it is not spinning. A string is wrapped tightly around the disk, and you pull on the string with a constant force of 42 N through a distance of 0.9 m. Now what is the angular speed

Answer 1

The angular speed is 23.24 rad/s.

Step-by-step explanation:

Given;

mass of the disk, m = 7 kg

radius of the disk, r = 0.2 m

applied force, F = 42 N

distance moved by disk, d = 0.9 m

The torque experienced by the disk is calculated as follows;

τ = F x d = I x α

where;

I is the moment of inertia of the disk = ¹/₂mr²

α is the angular acceleration

F x r = ¹/₂mr² x α

The angular acceleration is calculated as;

`\alpha = (2Fr)/(mr^2) \\\\\ \alpha = (2F)/(mr)\\\\\alpha = (2 * 42 )/(7 * 0.2) \\\\\alpha = 60 \ rad/s^2`

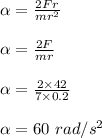
The angular speed is determined by applying the following kinematic equation;

`\omega _f^2 = \omega_i ^2 + 2\alpha \theta`

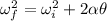
initial angular speed, ωi = 0

angular distance, θ = d/r = 0.9/0.2 = 4.5 rad

`\omega _f^2 = 2\alpha \theta\\\\\omega _f = √(2\alpha \theta) \\\\\omega _f = √(2 * 60 * 4.5) \\\\\omega _f = 23.24 \ rad/s`

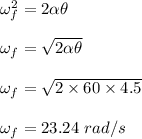
Therefore, the angular speed is 23.24 rad/s.