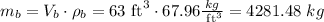Step-by-step explanation
From the statement, we have the following objects.
1) A water tank made of polyethylene with:
• height h = 8 ft,
,
• width or diameter d = 5 ft,
,
• thickness t.
2) A concrete base with:
• base b = 9 ft,
,
• width w = 7 ft,
,
• height h = 1 ft.
We also know that:
• the density of polyethylene is ρₚ = 0.900 g/cm³,
,
• the density of concrete is ρc = 2.4 g/cm³.
We convert the densities to g/ft³:

1) Volume of the water tank
Using the data from the water tank, we make the following diagram:
The volume of the water thank is given by:
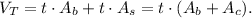
Where:
• Ab = area of the base,
• Ac = area of the curved side,
,
• t = thickness of the tank.
The area of the base is:
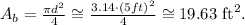
The area of the curved side is:
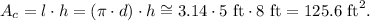
Replacing th values of Ab and Ac in the formula above, we get thevolume of the tank
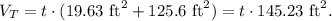
The mass of the tank is given by:
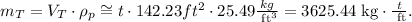
By replacing the value of the thickness, we get the mass of the tank.
2) Voume of the base
The concrete base is a rectangular prism. Its volume is given by:
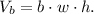
Replacing the values of b, w and h, we get:
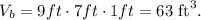
The mass of the base is:
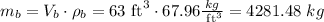
Answer
• The ,mass of the water tank, is:
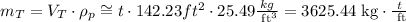
Replacing the value of the thickness t (in ft), we get the mass.
• The ,mass of the concrete base, is: