Given data:
* The original length of the rope is L = 3.72 m.
* The area of cross-section of rope is,
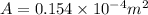
* The Young's modulus of rope is,

* The mass of the load is,
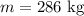
Solution:
The weight of the load is,
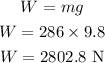
Young's modulus of the rope in terms of the weight, area, and the original length of the rope is,
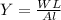
where l is the change in the length,
Substituting the known values,
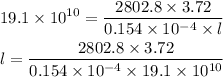
By simplifying,

Thus, the increase in the length of the rope is 3.54 mm.