Question

Two linear functions are represented below , f(x) by a table , and g(x) by a graph .which function has the greater rate of change

Answer 1

The rate of change of a function is equal to the derivative of that function.

So, we can proceed by finding the derivative of functions f and g. The one with the greatest derivative will then have the greatest rate of change.

Function f(x).

From the table, we can observe that

x=0-->f(x)=4 and x=5-->f(x)=7

Let's see if this function is a line. Remember that to define a line we only need two points, we can take (x,f(x))=(0,4),(5,7) so as to facilitate the solution.

`\begin{gathered} (0,4),(5,7) \\ f(x)-f(x_1)_{}=\frac{(f(x_2)_{}-f(x_1)_{})}{(x_2-x_1)}(x-x_1) \\ \Rightarrow f(x)-4=((7-3))/((5-0))(x-0) \\ \Rightarrow f(x)-4=(4)/(5)x \end{gathered}`

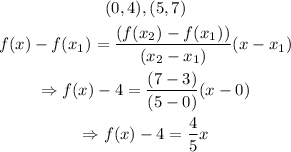
Now, let's verify that this equation models the information displayed in the table:

x=-10-->f(x)=-2